Rikimarox2
He/Him- 8,004
- 4,546
Ah so it's just unquantifiable?
Man, that sucks.
Man, that sucks.
-
This forum is strictly intended to be used by members of the VS Battles wiki. Please only register if you have an autoconfirmed account there, as otherwise your registration will be rejected. If you have already registered once, do not do so again, and contact Antvasima if you encounter any problems.
For instructions regarding the exact procedure to sign up to this forum, please click here. -
We need Patreon donations for this forum to have all of its running costs financially secured.
Community members who help us out will receive badges that give them several different benefits, including the removal of all advertisements in this forum, but donations from non-members are also extremely appreciated.
Please click here for further information, or here to directly visit our Patreon donations page. -
Please click here for information about a large petition to help children in need.
Official Calculations Discussion Thread
- Thread starter Antvasima
- Start date
-
- Tags
- vs battles wiki
- 5,871
- 3,762
How would I calculate lifting strength from ripping off and shattering thick steel constraints?
Here are the feats I plan to calculate:
1
2
I was using the chain-breaking formula without accounting for hollowness since it's not hollow at all, but realized partway through that there may be a different method in this situation (Chain breaking formula resulted in high Class M Lifting Strength).
Here are the feats I plan to calculate:
1
2
I was using the chain-breaking formula without accounting for hollowness since it's not hollow at all, but realized partway through that there may be a different method in this situation (Chain breaking formula resulted in high Class M Lifting Strength).
- 17,626
- 13,162
problem is, we don't know the magnitude.
I'd say at least a V Mercalli scale
- 16,171
- 12,705
just making stuff shake is Mag 4 I believe.
- 17,626
- 13,162
mag 4 is only like minor shaking of plates and shiz. Mag 5 is when trees start shaking and bushes and stuff.
- 5,871
- 3,762
Bump? (Not sure how things work here. Just wanted to make sure this gets attention since I've had a blog open since yesterday)
- 32,835
- 38,111
Hmmmmm, calculate the main area at which it was broken off? It'd be the thickness and the width of the metal parts. And then multiply it with the tensile strength of the material.
- 5,871
- 3,762
I believe it broke off on both sides (and down the middle it seems) for the first one, and in the second one (the rounded metal beam) it appeared to shatter all over the place rather than just one part.
By area, do you mean the cross-sectional area that was broken? If so, do I still multiply it by 1e+6 and the tensile strength? (Also if we only measured the break by the part that was broken, would it be better to only measure the chains that were broken rather than the whole chain since chains usually break in half or into a few pieces? Because fro what I've seen people usually take the cross-section of the whole chain)
- 3,654
- 369
Just a passing thought, what are people's best thoughts on measuring the reaction/combat speed of intercepting a multi-simultaneous projectiles attack one by one in a near instant?
like taking down multiple projectiles that are coming at you all at once, at the same speed and the same distance, and you take them down one by one at such speed that it looks to be almost like they were all stopped at once or hit a wall at the same time.
I have at least two methods to try out
First method of calculating is based on the regular reaction/combat speed of intercepting incoming simultaneous projectile attack.
like taking down multiple projectiles that are coming at you all at once, at the same speed and the same distance, and you take them down one by one at such speed that it looks to be almost like they were all stopped at once or hit a wall at the same time.
I have at least two methods to try out
First method of calculating is based on the regular reaction/combat speed of intercepting incoming simultaneous projectile attack.
- first you find the distance between the projectiles and the interceptor by the time both move
- You divide the two
- then multiply the speed of one of the projectiles
- then you multiply the others by the total number
- and (This one is more tricky) in order for it to not appear to be instantaneous or shot multiple projectiles all at once to intercept (otherwise you get ridiculously high speed) you would need to multiply the time/distance delay between each shot and then divide by the original speed result.
- first you would get the # of attacks and * by 60 seconds to get actions per minute.
- Then to find the velocity (meters per minute), you must multiply the frequency (APM) by the distance (meters) in question.
- Then you would multiply by the number of projectiles to the total velocity to cover all the distance.
- and (like the one above) in order for it to not appear to be instantaneous or shot multiple projectiles all at once to intercept (otherwise you get ridiculously high speed) you would need to multiply by the time/distance delay between each shot.
Last edited:
- 32,835
- 38,111
Then the area times two, since you're breaking two copies of the same thing
Area must be in m^2 and the tensile strength must be in Pascal.
Just the part where it breaks instead of applying it to the whole thing.
Suppose a rectangular meal beam is torn off. Its length is 20 m, width 0.07 m and thickness is 0.035 cm. The ripping was a vertical yank. Here the area affected would be the width times thickness, basically a super small rectangle.
Another example. A rod of the same length of 20 m has a diameter of 0.04 m. Area affected would be the cross-sectional area of the rod AKA its ends, so circle: pi * r^2.
Once you get the area, just multiply it with the pascal value and boom. LS value obtained.
Be careful tho, sometimes it may get crazy insane results.
Rikimarox2
He/Him- 8,004
- 4,546
Ello! I'm back yet again!
I have a few questions, is it possible to calc these feats, and how?
The first one: The boss makes a huge beam.
The second one: The boss makes... flaming tornados or smth?
The third one: At the beginning of the fight, the boss causes an eclipse.
And the last one: The boss makes these black-hole looking things. I assume this one would just be black hole creation? Especially since it can apparently be frozen (Granted, not by the MC even if he has ice manip) They stated to be Black-Holes, fyi.
I have a few questions, is it possible to calc these feats, and how?
The first one: The boss makes a huge beam.
The second one: The boss makes... flaming tornados or smth?
The third one: At the beginning of the fight, the boss causes an eclipse.
And the last one: The boss makes these black-hole looking things. I assume this one would just be black hole creation? Especially since it can apparently be frozen (Granted, not by the MC even if he has ice manip) They stated to be Black-Holes, fyi.
- 3,654
- 369
I have recently got a better understanding about perception and reaction speeds for characters and projectiles and how to calculate simultaneous attacks, This question is about finding the velocity from frequency.
The reason for this is when trying to do a high-low end with distance which gets weird results.
I want to ask for those capable, do you measure distance from the point of view of the character, or from the point of view of the incoming attack?
For finding volume from frequency, you would need to multiply the distance in meters from frequency which is rotations per minute to get velocity which is meters per minute.
vsbattles.fandom.com
which there is a document about it as well:
Can anyone help with this?
The reason for this is when trying to do a high-low end with distance which gets weird results.
I want to ask for those capable, do you measure distance from the point of view of the character, or from the point of view of the incoming attack?
For finding volume from frequency, you would need to multiply the distance in meters from frequency which is rotations per minute to get velocity which is meters per minute.
Ishida Reaction
vsbattles.fandom.com
which there is a document about it as well:
Can anyone help with this?
Rikimarox2
He/Him- 8,004
- 4,546
Bumpo
- 16,171
- 12,705
It looks like he's reacting to the arrow yeah. Mind you it'd give relatively low results since he barely moved the shield
Rikimarox2
He/Him- 8,004
- 4,546
Bump
CuteAnimeNekoGirl99
She/Her- 3,209
- 1,706
- 7,677
- 1,373
The increased mass would lead to a loss in momentum due to conservation of energy, but it wouldn’t all be gone due to that.
- 3,654
- 369
Okay, so what kind of feat would I get if you utilize perpetual momentum?
- 4,699
- 5,470
How can this disaster be calculated?
Context: the peninsula was shattered in a disaster, caused by the cataclysmic eruption of a chain of volcanic mountains that ringed Valyria. Vast amounts of land fell into the sea, with the surviving remnants becoming islands separated by water.
Context: the peninsula was shattered in a disaster, caused by the cataclysmic eruption of a chain of volcanic mountains that ringed Valyria. Vast amounts of land fell into the sea, with the surviving remnants becoming islands separated by water.
- 32,835
- 38,111
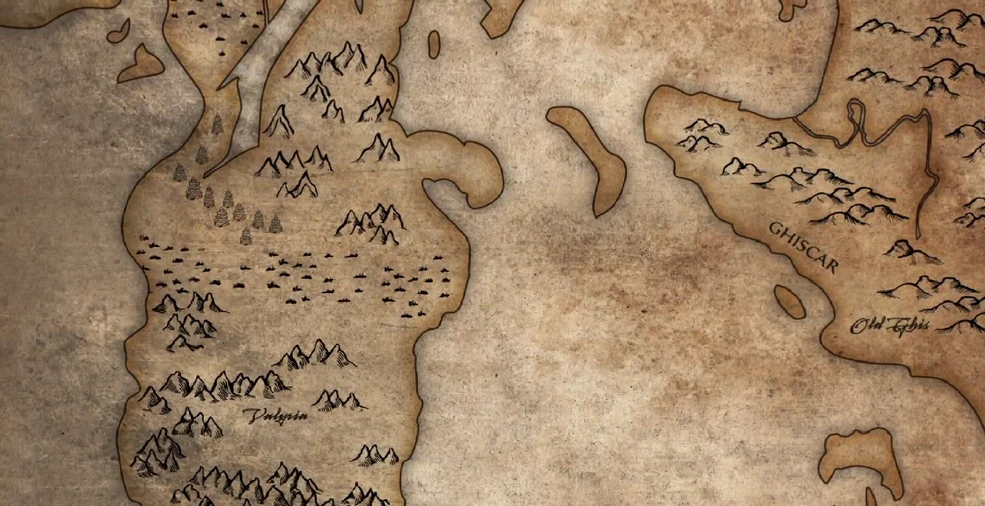
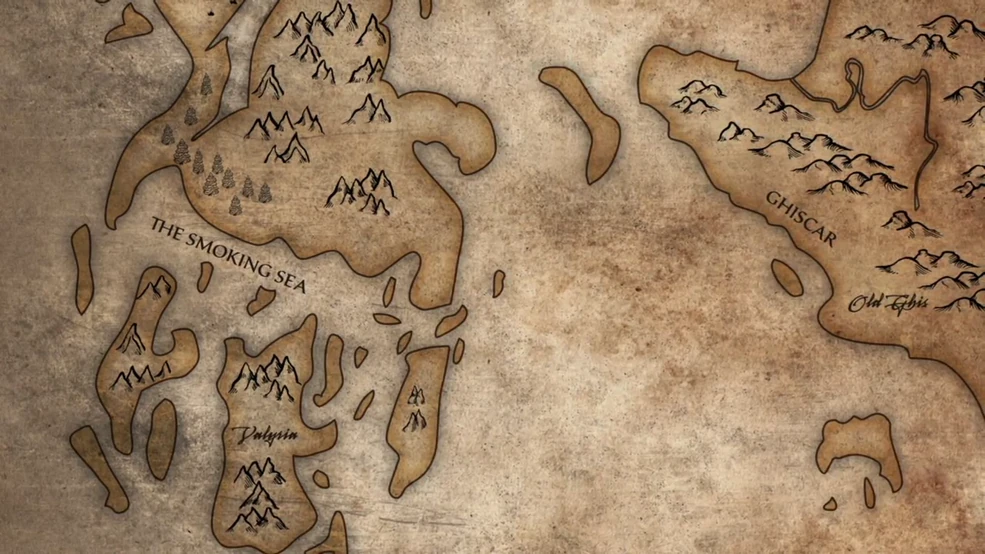
You could compare it to the nearest comparable IRL distaster that had similar results.How can this disaster be calculated?
Context: the peninsula was shattered in a disaster, caused by the cataclysmic eruption of a chain of volcanic mountains that ringed Valyria. Vast amounts of land fell into the sea, with the surviving remnants becoming islands separated by water.
- 32,835
- 38,111
No need for destruction values at that scale, just straight up go with GBE if the planet can no longer reform.
DenLitz
He/Him- 309
- 143
Can you explain/elaborate why only use the GBE Formula, I want to have my own opinion/understanding about this, not just follow along without understanding the meaning/explanation
- 32,835
- 38,111
Because it's effectively simpler to use as you're basically overpowering gravity from being able to reform the planet, whereas using destruction values you have to account for the liquid and molten iron being dissipated as well (And their dissipation speed), not only that you also have to assume the water itself being vaporized as a result of such an explosion. It'd be easier if it were something like the moon (Which is mostly rock) and assuming it hadn't completely escaped gravity's hold, but with Earth and other similar planets, this can get really tricky simply due to the material composition (Can't shatter something that's already molten), so GBE is used when we're absolutely certain that all of the Earth is blasted beyond being able to reform back into a ball due to gravity, thus at that point and scale it's basically counterintuitive to use the normal destruction values. And as you can see, we also don't use destruction values for gas giants or stars, gas giants as a whole have no solid surface in them and their destruction is determined by gravitational binding energy. Same with stars, like our Sun.
Also if you have an on-screen feat of planets being busted at high speeds with a proper timeframe, just use KE at that point.
Last edited:
DenLitz
He/Him- 309
- 143
So combining Destruction Result & GBE to get this Feat result is wrong right? i guess he destroyed the planet = Destruction, and not reunited into one = GBE, and the solution is Destruction Value + GBE Value.
- 32,835
- 38,111
More like there's no need to use destruction values in the first place at that scale (I'd argue it doesn't account for a lot of the other non-solid parts of the planet, like the mantle and the outer core and the oceans and their dispersion speed, if that makes sense), if the planet is blasted beyond any chance of reforming and coming back as a gravitationally bound body, GBE is more than enough.
Once again, read above. Destruction values at this scale becomes effectively unnecessary. If the planet can no longer come back and reform, the solution is to just use GBE.
DenLitz
He/Him- 309
- 143
Ok I get it, thanks for the response
DenLitz
He/Him- 309
- 143
I've seen in one thread @KLOL506 it said "J/Cc used for Fragmentation comes from MPa units" something like that, what I want to ask is how the Staff/Calc Group here converts MPa to J/Cc? I'd like a fairly clear answer (like the formula for converting MPa to J/Cc, or maybe there's a note to remember for converting MPa to J/Ccc, something like that), because I'm interested in calculations, and I hope I can also be a Calc Group in here
- 32,835
- 38,111
MPa and J/cc are practically the same units.
As for conversion, here's an example (Credits to Firestorm808 for showing the conversion method itself):
Low End Shear Strength of Concrete (Since we use shear strength for frag) as per our Destruction Values chart = 6 MPa = 6*10^6 N/m^2
1 Joule = 1 Newton * 1 Meter
1 Joule / 1 Meter = 1 Newton
6*10^6 Newtons = 6*10^6 J / Meter
6*10^6 J / Meter / Meter^2 = 6*10^6 J / Meter^3 = 6 J/cm^3 = Fragmentation
In essence, 1 MPa is 1 J/cm^3.
DenLitz
He/Him- 309
- 143
I see, thanks to the Calc Group for responding
Similar threads
- Sticky
- Featured
